Sunday, November 11, 2018
Overlapping Shapes
Original graph: https://www.desmos.com/calculator/jvayvgkw1o
Description: A grid of hexagons with dimensions controlled by a slider.
Original graph: https://www.desmos.com/calculator/cuw7dcdvwo
Description: Nine right triangles with midpoints of hypotenuses forming a circle, rotating about the midpoints.
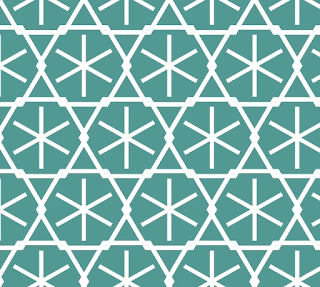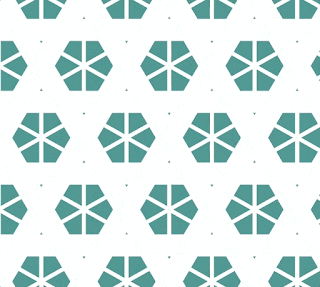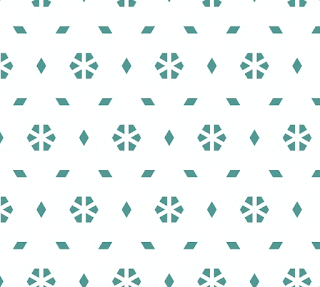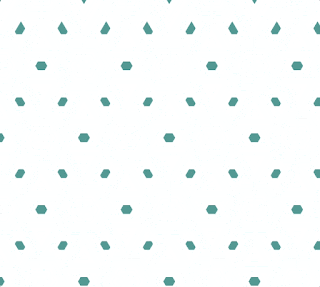
Spoked Hexagons
I've been playing around lately with the art of overlapping shapes. There is much to explore!
Each of these animations includes an image of a hexagon from Keynote (no fill, white border) repeated to form a grid of hexagons. The spokes at the centers of the hexagons are also images from Keynote.
Animations were created using Giphy Capture, and are based on this Desmos graph.
Friday, September 14, 2018
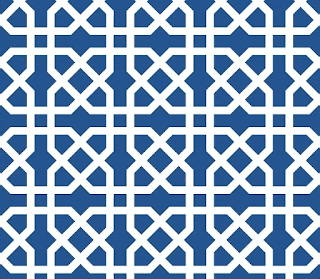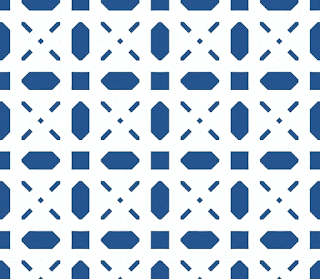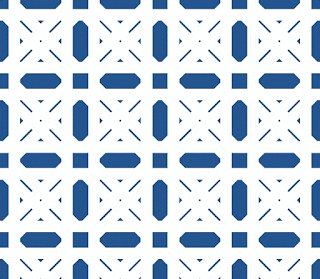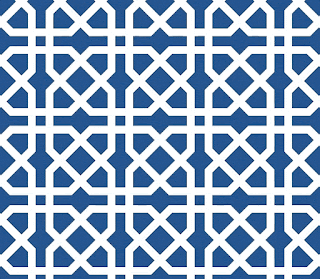
Desmos Islamic Art
All of these images were made using shapes from Keynote and the Desmos graphing calculator.
Some other favorites. I've called these brick patterns.
Thursday, August 16, 2018
Desmos Panda Project
This panda was made in Desmos using a few images of black, white, and pink circles that I made in Keynote. I made lists to set their centers, dimensions, and angles. I also graphed an ellipse for the head and two parametric circles for the mouth. I thought about symmetry a lot while working on this graph.
Note: I am interested in exploring what a Desmos art/animation course would look like for personal reasons, but also I am interested in exploring what it would look like because I'd love to see a course like this offered at the high school or college level as a math elective.
My elementary aged kids and nephew asked me if I was doing work when I made this (I work at Desmos). I told them I was doing it for fun, and that I thought maybe it would be cool if they worked on a similar project in their math class one day. They all seemed to like the idea and sat around me engaging in a discussion about the math I was using.
Question: What mathematics would students need to know before working on the panda project?
Note: I am interested in exploring what a Desmos art/animation course would look like for personal reasons, but also I am interested in exploring what it would look like because I'd love to see a course like this offered at the high school or college level as a math elective.
Tuesday, August 14, 2018
Desmos Animation 101: Image Orientation
Play with this graph. How does each variable change the arrow images?
I made the graph above to explore how the following function controlled the angle of the leaves in Suzanne von Oy's The Tree of All Seasons.
Here's a related challenge: https://www.desmos.com/calculator/3seqxrpsbi
Can you move the leaves to tree 2 and adjust the angle of the leaves so they are facing away from the center of the tree?
Question: What are other uses of this function that people might explore before working on the The Tree of All Seasons problem?
Note: I am interested in exploring what a Desmos art/animation course would look like for personal reasons, but also I am interested in exploring what it would look like because I'd love to see a course like this offered at the high school or college level as a math elective.
I made the graph above to explore how the following function controlled the angle of the leaves in Suzanne von Oy's The Tree of All Seasons.
Here's a related challenge: https://www.desmos.com/calculator/3seqxrpsbi
Can you move the leaves to tree 2 and adjust the angle of the leaves so they are facing away from the center of the tree?
Question: What are other uses of this function that people might explore before working on the The Tree of All Seasons problem?
Note: I am interested in exploring what a Desmos art/animation course would look like for personal reasons, but also I am interested in exploring what it would look like because I'd love to see a course like this offered at the high school or college level as a math elective.
Sunday, August 12, 2018
Peacock Project
Saturday, August 11, 2018
Desmos Animation 101: Part 2
Suzanne von Oy shared a graph titled The Tree of All Seasons awhile back as one of her #graphjam submissions. It's mesmerizing at the very least, and left quite a few of us wishing for a Desmos art course so Suzanne can teach us her magic.
I look forward to this course, but as I dove into the graph, I found myself quickly overwhelmed. So many variables and functions. I wasn't sure where to start with understanding the various parts. This left me wondering what the different parts are of the Desmos art course that will allow me to build this (and any) awesome graph.
So where would the course start? There are quite a few things going on just with the flowers in The Tree of All Seasons graph.
1. The flowers are all different sizes. Suzanne uses exactly one image to render all of the flowers, so I'm curious as to how she made them all different sizes.
2. The flowers start growing at different times. They start falling at different times. They start shrinking at different times. I imagine these are all related somehow.
3. Once the flowers shrink down to size zero, they stay at that size throughout the animation.
In my first post, I looked at the way in which we can control the size of the flowers using a function. In this post, I want to look at the way in which we can control the timing for which the flowers bloom and shrink.
I stared at this line of Suzanne's graph for a really long time.
After breaking it down and figuring out that the first expression (in parenthesis) assigns each flower its length and width depending on its y-value, I looked at the second expression and thought about what it does:
If I graph this I see the following:
Note: I am interested in exploring what a Desmos art/animation course would look like for personal reasons, but also I am interested in exploring what it would look like because I'd love to see a course like this offered at the high school or college level as a math elective.
I look forward to this course, but as I dove into the graph, I found myself quickly overwhelmed. So many variables and functions. I wasn't sure where to start with understanding the various parts. This left me wondering what the different parts are of the Desmos art course that will allow me to build this (and any) awesome graph.
So where would the course start? There are quite a few things going on just with the flowers in The Tree of All Seasons graph.
1. The flowers are all different sizes. Suzanne uses exactly one image to render all of the flowers, so I'm curious as to how she made them all different sizes.
2. The flowers start growing at different times. They start falling at different times. They start shrinking at different times. I imagine these are all related somehow.
3. Once the flowers shrink down to size zero, they stay at that size throughout the animation.
In my first post, I looked at the way in which we can control the size of the flowers using a function. In this post, I want to look at the way in which we can control the timing for which the flowers bloom and shrink.
I stared at this line of Suzanne's graph for a really long time.
After breaking it down and figuring out that the first expression (in parenthesis) assigns each flower its length and width depending on its y-value, I looked at the second expression and thought about what it does:
If I graph this I see the following:
This graph assigns a "size" to the flowers depending on time. It essentially says the flowers will grow from size 0 to full size (or size 1) in a certain amount of time. Then the flowers will stay full sized for a different amount of time, after which they will shrink down to size 0 and stay that size throughout the animation. Super useful!
Question: What are other uses of this function that people might explore before working on the The Tree of All Seasons problem?
Note: I am interested in exploring what a Desmos art/animation course would look like for personal reasons, but also I am interested in exploring what it would look like because I'd love to see a course like this offered at the high school or college level as a math elective.
The Tree of All Seasons/Desmos Art Class
Note: This was originally posted on the Making Math Visual blog. Crossposting here and moving these Desmos art class posts to Math Art Animation 101 blog.
Suzanne von Oy shared a graph titled The Tree of All Seasons awhile back as one of her #graphjam submissions. It's mesmerizing at the very least, and left quite a few of us wishing for a Desmos art course so Suzanne can teach us her magic.
I look forward to this course, but as I dove into the graph, I found myself quickly overwhelmed. So many variables and functions. I wasn't sure where to start with understanding the various parts. This left me wondering what the different parts are of the Desmos art course that will allow me to build this (and any) awesome graph.
So where would the course start? There are quite a few things going on just with the flowers in The Tree of All Seasons graph.
1. The flowers are all different sizes. Suzanne uses exactly one image to render all of the flowers, so I'm curious as to how she made them all different sizes.
2. The flowers start growing at different times. They start falling at different times. They start shrinking at different times. I imagine these are all related somehow.
3. Once the flowers shrink down to size zero, they stay at that size throughout the animation.
I started exploring question 1, or how I can make all of the flowers different sizes using just one copy of the flower image. You can make multiple copies of an image by using a list to define the center of the image. In this case we could use (x_1,y_1) to define the center of the flowers since the centers are all listed in the table.
I made some efforts to apply my previous knowledge of animating images in the calculator and failed very quickly. My next move was to dig into the function Suzanne uses to control the dimensions of the flowers. Even that was tough, but I found some success by looking at the function one piece at a time. Here's the first piece:
This is one way we can define the length and width of the flower image. At its max, the value is something like 0.833, and at its min, the value is 0.5. I imagine Suzanne started with her minimum value in mind and played around with the numbers in the mod function to find a nice maximum value. (Suzanne, let us know if you thought of this in a different way).
Here's a graph with just five flowers. The dimensions are controlled using the function above, which depends on the y-variable. Turn on the movable points and move them around to see how it works.
Question: What are other uses of this function that people might explore before working on the The Tree of All Seasons problem?
Note: I am interested in exploring what a Desmos art/animation course would look like for personal reasons, but also I am interested in exploring what it would look like because I'd love to see a course like this offered at the high school or college level as a math elective.
Suzanne von Oy shared a graph titled The Tree of All Seasons awhile back as one of her #graphjam submissions. It's mesmerizing at the very least, and left quite a few of us wishing for a Desmos art course so Suzanne can teach us her magic.
I look forward to this course, but as I dove into the graph, I found myself quickly overwhelmed. So many variables and functions. I wasn't sure where to start with understanding the various parts. This left me wondering what the different parts are of the Desmos art course that will allow me to build this (and any) awesome graph.
So where would the course start? There are quite a few things going on just with the flowers in The Tree of All Seasons graph.
1. The flowers are all different sizes. Suzanne uses exactly one image to render all of the flowers, so I'm curious as to how she made them all different sizes.
2. The flowers start growing at different times. They start falling at different times. They start shrinking at different times. I imagine these are all related somehow.
3. Once the flowers shrink down to size zero, they stay at that size throughout the animation.
I started exploring question 1, or how I can make all of the flowers different sizes using just one copy of the flower image. You can make multiple copies of an image by using a list to define the center of the image. In this case we could use (x_1,y_1) to define the center of the flowers since the centers are all listed in the table.
I made some efforts to apply my previous knowledge of animating images in the calculator and failed very quickly. My next move was to dig into the function Suzanne uses to control the dimensions of the flowers. Even that was tough, but I found some success by looking at the function one piece at a time. Here's the first piece:
This is one way we can define the length and width of the flower image. At its max, the value is something like 0.833, and at its min, the value is 0.5. I imagine Suzanne started with her minimum value in mind and played around with the numbers in the mod function to find a nice maximum value. (Suzanne, let us know if you thought of this in a different way).
Here's a graph with just five flowers. The dimensions are controlled using the function above, which depends on the y-variable. Turn on the movable points and move them around to see how it works.
Note: I am interested in exploring what a Desmos art/animation course would look like for personal reasons, but also I am interested in exploring what it would look like because I'd love to see a course like this offered at the high school or college level as a math elective.
Subscribe to:
Comments (Atom)